Errata, Comments
1. Weighing operator spectra. Indiana Univ. Math. J.
21 (1971) 61--80. [with J. Ernest and S.-G. Lee] MR 54#5884
errata
Page 61, first line of text. After "nonseparable" insert "Hilbert"
Page 61, line 4 from bottom. "dided" should be "sided".
Page 66, line 2 from bottom. "H" should be in script font.
Page 73, line 21. "p int" should be "point".
Page 75, line 5 from bottom. Replace "infinite Hilbert spaces of dimension" by
"Hilbert spaces of infinite dimension".
Page 76, line 10. Replace "the diagonal" by "a diagonal.
Page 76, line 12. Replace "the diagonal" by "a diagonal.
Page 78, line 12. Replace "Theorem 4.3" by "Corollary 3.3".
Page 79, line 8. Replace "An operator" by "A Hermitian operator".
Page 79, reference 15. Period after "Ind"
2. Perfect n-sequences for n, n+1, and
n+2. Fibonacci Quart. 10
(1972) 377--380. MR 46#7152
errata
Page 392, second row of the table. The second 1 should be n+1
4. The class of topological spaces is equationally definable.
Algebra Universalis 3 (1973) 139--146. MR 51#3026
errata
Page 140, line 19. Italic x should be boldface.
Page 140, line 9 from bottom. The first script S should be Fraktur.
Page 141, line 17. Replace "inductively" by "as follows".
Page 142, line 6. Delete "reflective". In the second "Alg", italic
A should be boldface.
Page 143, lines 22 and 23. D in subscript should be italic (twice).
Page 144, in (K3). Coproduct should be product (Pi should not be inverted).
Page 144, in (K4). First subscript D should be superscript. Product
should be coproduct (Pi should be inverted).
Page 146, reference [2]. "Birkhiff" should be "Birkhoff".
5. Pointwise convergence in terms of expectations. Z.
Wahrscheinlichkeitstheorie und Verw. Gebiete 30 (1974) 17--26.
[with D. G. Austin and A. Ionescu Tulcea] MR 50#11402
errata
Page 19, line 10 from bottom. Display should end with
![[formula]](E05a.gif)
8. Disintegration of measures and the vector-valued Radon-Nikodym
theorem. Duke Math. J. 42 (1975)
447--450. MR 54#507
errata
Page 448, line 6. last comma should be period.
Page 448, line 18. Replace "to" by "be".
comments
-
See Bourbaki, Intégration, Chap. IX,
Sec. 5, exercise 10c, p. 109. Is this RNP for probability measures on
X, where X is a Souslin space?
- Similar theorems:
- J. Hoffman-Jorgensen, Math Scand.
28 (1971) 257--264
- M Valadier, C. R. Acad. Sci. Paris
267 (1973) A33--35.
- J. St.-Pierre, Ann. Inst. H. Poincaré
11 (1975) 275--286. (infinite measures)
- Generalized (pseudo-compact sets replacing compact)
- A. G. A. G. Babiker & W. Strauss, The pseudostrict topology on
function spaces. Rend. Ist. Matem. Univ. Trieste
14 (1982) 99--105.
10. Amarts: a class of asymptotic martingales, A. Discrete
parameter. J. Multivariate Anal. 6 (1976) 193--221. [with L.
Sucheston] MR 54#1368
errata
DVI file (2 K)
PostScript file (100 K)
comments
-
Chacon's inequality:
-
R. Chen, A simple proof of a theorem of Chacon.
Proc. Amer. Math. Soc. 60 (1976) 273--275.
-
other versions equivalent to "amart"
-
Chatterji, Manuscr. Math. 1971
-
Lamb, Canad. J. 1973
11. The Riesz decomposition for vector-valued amarts. Z.
Wahrscheinlichkeitstheorie und Verw. Gebiete 36 (1976) 85--92.
[with L. Sucheston] MR 54#1369
errata
Page 89, line 3 from bottom. p should be < 2.
12. Extremal integral representations. J. Functional Anal.
23 (1976) 145--161. MR 55#8753
errata
Page 160, line 10. "measure" should be "measures".
comments
-
Question page 149: affirmative answer, D. Fremlin, June 1981.
-
Lemma 1.3: see also
-
Haydon, An extreme point criterion... Quart. J. Math.
27 (1976) 379--385; Lemma 3.1, p. 382.
-
Talagrand constructed an example with lots of maximal measures, but
no extreme points.
-
This monad is described in:
-
M. Giry, A categorical approach to probability theory.
Lecture Notes in Math. 915 (1982) 68--85.
-
A space PX of measures is defined for any topological space X by the universal
mapping property in:
-
Leger & Soury, J. Math. Pures et Appl.
50 (1971) 363--425.
-
Example in Section 6: For a related example (?) see
-
B. Rodrigez-Salinas, Un converse sin representacion integral extremal, 1983
-
Example in Section 6: Is there such a counterexample in every nonseparable
Banach space? [Suárez, Fremlin, Talagrand]
13. Noncompact simplexes in Banach spaces with the Radon-Nikodym
property. J. Functional Anal. 23 (1976) 162--176. [with R. D.
Bourgin] MR 55#8754
errata
DVI file (3 K)
PostScript file (130 K)
14. Measurable weak sections. Illinois J. Math.
20 (1976) 630--646. MR 54#7734
errata
Page 633, line 3. "[11, p. 144]" should be "[12, p. 144]"
Page 635, lines 10-11. Omit (b) and (c).
Page 638, line 6. "Thus" to end of proof: Correction in the comments document below.
Page 638, line 9 from the bottom. "F" should be in Fraktur font.
Page 639, line 14. "E" should be in Fraktur font.
Page 644, line 2. "A" should be in italic.
Page 644, line 6. This need not define a lifting! It may not be equivalent
to f.
comments
DVI file (3 K)
PostScript file (45 K)
15. Amarts: a class of asymptotic martingales, B. Continuous
parameter. J. Multivariate Anal. 6 (1976) 572--591. [with L.
Sucheston] MR 55#4370
errata
Page 578, line 8. "x" should be capitalized
Page 582, line 10 from bottom. Following the display, insert box for end of proof.
Page 588, line 7. Last two times, "t" should be "s".
16. Measurability in a Banach space. Indiana Univ. Math. J.
26 (1977) 663--677. MR 58#7081
errata and comments
DVI file (6 K)
PostScript file (220 K)
18. Martingales in the limit and amarts. Proc. Amer. Math. Soc.
67 (1977) 315--320. [with L. Sucheston] MR 58#7832
comments
L. H. Blake, Every amart is a martingale in the limit. J.
London Math. Soc. 18 (1978) 381--384.
Yamasaki, Another convergence theorem for martingales in the limit.
Tohoku Math. 33 (1981) 555--559.
19. On the Radon-Nikodym property and martingale convergence. In:
Vector Space Measures and Applications II, R. M. Aron and
S. Dineen (editors), Lecture Notes in Mathematics 645,
Springer-Verlag, 1978. pp. 62--76. MR 80e:28012
errata
Page 65, line 5 from bottom. Insert "open" before "neighborhood".
Page 66, last line. Delete the first right parenthesis ")"
Page 67, line 10 from bottom. Subscript c should be b.
Page 67, line 7 from bottom. X should be C.
Page 68, line 14. Insert minus sign at beginning of equation.
Page 69, line 15 from bottom. "merely are" should be "merely an"
Page 69, line 12 from bottom. "at least" should be "a least" (twice).
Page 70, line 15. "chows" should be "shows".
Page 70, line 4 from bottom. See the first comment, below.
Page 74, line 13. The first phi should have a prime.
comments
-
Theorem 2.4 is not correct as stated. It can be repaired by changing
condition (b') to: The least upper bound in
![[formula]](E19a.gif) of
a chain in
of
a chain in ![[formula]](E19b.gif) lies in
lies in
![[formula]](E19b.gif) . Now Proposition 1.5 will apply as claimed
to show (b') implies (b). A similar change should be made in (c').
. Now Proposition 1.5 will apply as claimed
to show (b') implies (b). A similar change should be made in (c').
-
See also:
-
D. A. Edwards, On the existence of probability measures with
given marginals. Ann. Inst. Fourier Grenoble
28 (1978) 53--78.
- "Converse" of 2.7
-
E. G. F. Thomas, Oberwolfach, Lecture Notes in Math 794, page 497.
-
B. Fuchssteiner, An abstract disintegraion theorem.
Pacific J. Math. 94 (1981) 303--310.
- Strassen for a cone.
-
Pflug, The equivalence of the Bishop--de Leeuw and the Pigou--Dalton
order structures of measures. Riv. Mat. Sci. Econom.
Social. 2 (1979) no. 1, 71--75.
-
M. Neumann, On the Strassen disintegration theorem.
Archiv. der Math. 29 (1977) 413--420.
-
G. Winkler, Inverse limits need not exist in the category of
compact spaces and Feller kernels: a counterexample.
In: Proceedings of the
12th winter school on abstract analysis (Srni, 1984). Rend. Circ. Mat.
Palermo (2) 1984, Suppl. No. 5, 155--159.
- Example shows that analytic, bounded, convex does not imply t-convex in general.
-
H. Rosenthal, sub-simplexes of convex sets... Contemp.
Math. 85 (1989) 447--463
- Contains a (very) partial solution for Remark (2).
-
Elton & Hill, Fusions of a probability distribution.
20. Three cryptoisomorphism theorems. In: Studies in Foundations
and Combinatorics, G.-C. Rota (editor), Advances in Mathematics
Supplementary Studies 1, Academic Press, 1978. pp. 49--60. MR
80c:54001
errata
Page 59, line 16. P should be M.
21. Measurability in a Banach space, II. Indiana
Univ. Math. J. 28 (1979) 559--579. MR 81d:28016
errata and comments
DVI file (6 K)
PostScript file (250 K)
24. Liftings of functions with values in a completely regular
space. Proc. Amer. Math. Soc. 78 (1980) 345--349. [with
M.
Talagrand] MR 81g:46057
comments
-
A. Bellow coined the term lifting-compact for the spaces described
in this paper.
-
A. Bellow, Lifting compact spaces. In: Measure
Theory, Oberwolfach 1979,
pp. 233--253, Lecture Notes in Math. 794:
-
(a) another version of Example 3
(b) the concept is independent of the choice of H
(c) example for some vs. all liftings
-
an earlier related paper
-
C. Ionescu-Tulcea, Liftings for functions with values in a completely
regular space. Math. Ann. 187 (1970) 200--206
-
later papers
-
Babiker & Strauss, Lecture Notes 695
Babiker, Heller, & Strauss, J. Austral. Math. Soc.
(Ser. A) 41 (1986) 211--223
25. A long James space. In: Measure Theory, Oberwolfach 1979, D.
Kölzow (editor), Lecture Notes in Mathematics 749,
Springer-Verlag, 1980. pp. 31--37. MR 81k:46018
comments
![[formula]](E25a.gif) has the metric approximation property
has the metric approximation property
errata
Page 33, line 14. "norm 1" should be "norm at most 1"
Page 35, last display. The intersection sign should be a union
26. Asplund operators and a. e. convergence. J. Multivariate
Anal. 10 (1980) 460--466. MR 82f:47053
errata
Page 461, line 4. ![[formula]](E26a.gif) should be
should be
![[formula]](E26b.gif)
Intégration by N. Bourbaki.
Math. Intelligencer 3 (1981) 82--84.
errata
Page 83, column 2, line 18 from bottom. "It will" should be "I will".
28. Characterization of probability distributions based on a
generalized Rao-Rubin condition. Sankhya Ser. A 42 (1980)
161--169. [with C. R. Rao, R. C. Srivastava, S. Talwalker] MR
83d:62032
errata
Page 166, line 10. "unit mass at r" should be "unit mass at 1".
Page 166, line 11. Display should be ![[formula]](E28a.gif)
Page 167, line 7. Argument is multiplied by s
Page 167, line 11. Equality should be approximate (as in previous display).
Page 169, line 2. ![[formula]](E28b.gif)
Page 169, third display. ![[formula]](E28c.gif)
Page 169, fourth display. ![[formula]](E28d.gif)
29. Additive amarts.
Ann. Probab. 10 (1982) 199--206. MR 83a:60081
errata
Page 200, line 13. ![[formula]](E29a.gif)
Page 203, line 2 from bottom. Element sign missing at the end of the line.
comments
Other related references:
A. Millet, Convergence and regularity of strong submartingales.
Grillenberger & Krengel, Remark on strong submartingales and
the linear embedding of tactics. J. Multivariate
Analysis 11 (1981) 568--571.
32. An ordering for the Banach spaces. Pacific J. Math.
108 (1983) 83--98. MR 84k:46012
comments
DVI file (5 K)
PostScript file (200 K)
errata
Page 85, line 8, Y should be in Fraktur font.
Page 85, line 5 from bottom, X should be Fraktur font.
Page 95, line7, spelling "reflexive".
34. Topological properties of Banach spaces. Pacific J. Math.
115 (1985) 317--350. [with R. F.
Wheeler] MR 86e:46013
comments
-
Reference for Kunen example:
-
S. Negrepontis, Banach spaces and topology. In:
Handbook of Set-Theoretic Topology, Kunen & Vaughn (editors),
North-Holland, 1987.
-
2.1, topological characterization of Asplund space, due to Fitzpatrick. See:
-
Bourgin, Geometric Aspects of Convex Sets with the
Radon-Nikodym Property, Theorem 5.4.1.
-
Argyros, Odell, and Rosenthal, On certain convex subsets of $c_0$.
-
Examples of CPCP not equivalent to PCP, etc.
-
The question on p. 345 concerning 5.2 is answered on page 299 of:
-
Rosenthal, Weak*-Polish Banach spaces. J. Funct. Anal.
76 (1988) 267--316.
-
Generalization of 4.1: PCA implies "somewhat quasireflexive":
-
C. Finet, Subspaces of Asplund Banach spaces with the Point Continuity
Property.
-
Ghoussoub & Maurey, G-delta embeddings in Hilbert space.
J. Funct. Anal. 61 (1985) 72--97.
-
Cor. III.1: X RNP, X* separable iff the remainder is a countable union
of w* compact convex sets. (Converse of 4.13)
-
Cor II.1: X PC implies X is "somewhat separable dual". (Generalizes 4.1)
-
R. W. Hansell, Descriptive sets and the topology of non-separable Banach
spaces.
errata
Page 322, line 19. Replace "Polish" by "complete metric".
Page 329, line 12 from bottom. Replace "obunded" by "bounded".
Page 332, display. Replace "daim" by "diam".
Page 322, line 5 from bottom. Replace semicolon by period.
Page 338, line 7. After "hence weakly Lindelof" add "[52]".
Page 339, first display. Delete absolute value bars (4 of them).
Page 340, line 12. Replace S** by ![[formula]](E34a.gif)
38. Analytic martingale convergence. J. Functional Anal.
69 (1986) 268--280. MR 88f:46046
errata
Upper and lower case Greek letters have been freely interchanged by the typesetter.
In particular, upper and lower case theta are the same.
Page 268, line 3 from bottom. Add parenthetical "(a.s.)" before "convergence properties".
Page 272, last line should end ![[formula]](E38a.gif)
Page 273, in two displays, the ratio of the r's should be inverted in the subscript of P.
39. Pi: difficult or easy? Math. Mag.
60 (1987) 141--150.
MR 89f:11170
errata
Page 142, line 8 from the bottom, "We will need...": The first > should be < .
comment
-
The following was published (as a letter to the editor) on page 252
of the same volume:
-
An alert reader (Genji Yoshino) has noted that there is an error
in one of the references in my article about pi in the June issue
of the Magazine. The correct reference is:
![[formula]](E39a.gif)
An additional reference that has recently been published is:
![[formula]](E39b.gif)
This contains (among much other material) a complete explanation of the
algorithms for pi depending on the arithmetic-geometric mean.
41. Kiesswetter's fractal has Hausdorff dimension 3/2.
Real Analysis Exchange 41 (1989) 215--223.
MR 90j:28003
comments
-
(February, 1989) The following note (too late to be added to the paper
itself) appeared in the following issue of the Exchange:
-
Tim Bedford has kindly sent me a copy of his Ph.D. thesis,
"Crinkly curves, Markov partitions and Dimension", University of Warwick,
September, 1984. It contains an evaluation of the dimension of Kiesswetter's
curve (by a different method).
(October, 1993) Roland Girgensohn (Simon Fraser University) obtained the
result in a more elementary way, using essentially the methods of
A. S. Kline, J. London Math. Soc. 20 (1945) 79--86.
errata
Last page, reference 7, correct spelling of "properties".
42. On maximal inequalities in Orlicz space.
In: Measure and Measurable Dynamics,
R. D. Mauldin, R. M. Shortt, C. E. Silva (editors),
Contemporary Mathematics 94, American
Mathematical Society, 1989. pp. 113--129. [with L. Sucheston]
MR 90k:46058
errata
Page 114, fourth display should be ![[formula]](E42a.gif)
45. A fractal puzzle. The Mathematical Intelligencer
13 (1991) No.3, 44--50. MR 92j:58062
comments
Color versions of some of the figures appear as Plates 15 and 16
in my book Measure,
Topology, and Fractal Geometry.
Three IFS programs for Macintosh. Amygdala
#24 (June 29, 1991) pp. 3--8.
errata
Page 4, left column, 10 lines from bottom. Insert right parenthesis
after "up to 1."
Page 4, right column, 9 lines form bottom. Change "Figure 3" to "Figure 4".
Page 4, right column, 6 lines form bottom. Change "Figure 3" to "Figure 5".
Page 5, Figure 4. Fourth picture is wrong.
48. Multifractal decompositions of digraph recursive
fractals. Proc. London Math. Soc. 65 (1992)
604--628. [with R. D. Mauldin]
MR 93h:28010
errata
Page 604, first line of the Introduction, "literature" with an extra "r".
49. Packing measure as a gauge variation. Proc. Amer.
Math. Soc. 122 (1994) 167--174. MR 94k:28009
errata
Page 170, Theorem (1.10), line 2 of the proof, the display should end
with ![[formula]](E49a.gif) (the superscript is missing).
(the superscript is missing).
50. Fine variation and fractal measures. Real Analysis
Exchange 20 (1995) 256--280. MR 96a:28012
comments
In the published version, the script and blackboard bold fonts
have been set as ordinary Roman. (Isn't TeX wonderful?)
The preprint
has the original fonts.
errata
Page 258, display in the middle of the page, Q should be
![[formula]](E50a.gif) (the set of rational numbers).
(the set of rational numbers).
Page 260, line 3, ? should be ![[formula]](E50b.gif) (the empty set).
(the empty set).
51. Carathéodory's outer measures: 80 years. Real Analysis
Exchange 20 (1995) 14--17
errata
Page 14, second paragraph:
"independence from the Ottoman Empire in 1820" (not 1920).
52. A fractal dimension estimate for a graph-directed iterated function system of
non-similarities. Indiana Univ. Math. J.
48 (1999) 429--448 [with Jeffrey Golds]
errata
Page 432, line 1. before "directed" insert "finite"
Page 434, line 8 from bottom. "2.1 Definition" should be "2.4 Definition"
Page 435, line 10. "(2.2)" should be "(2.5)"
Page 435, line 14. "(2.3)" should be "(2.6)"
Page 440, line 7. ![[beta]](E52a.gif) should be
should be
![[alpha ']](E52b.gif)
Page 446, reference [5]. "S. Drobot" should be "V. Drobot".
54. Packing measure in general metric space. Real
Analysis Exchange 26 (2001) 831-852.
errata
Page 833, line -5. Delete "¿".
Page 835, line -3. Delete "¿".
Page 843, line -3. "these case" should be "these cases".
Page 844, line 9. Delete "¿".
Page 848, line -5. "peripheral" should be "central".
56. Borel subrings of the reals. Proc. Amer. Math. Soc.
131 (2003) 1121-1129 [with Chris Miller]
errata
Page 1123, line -17. "an additive subgroup" should be "a subring".
Page 1126, line -10. "an additive subgroup" should be "a subring".
Page 1127, line 2. S should be τ.
Page 1127, line -7. "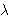 " should be "
" should be "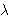
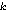 "
"
Page 1128, line 6. "an additive subgroup" should be "a subring".
Page 1128, line 14. "n-dimensional Haar measure coincides with Hausdorff measure"
should be "Haar measure coincides with n-dimensional Hausdorff measure".
Page 1129, line 7. "matrischer" should be "metrischer"
email
edgar@math.ohio-state.edu
![[formula]](E19a.gif) of
a chain in
of
a chain in ![[formula]](E19b.gif) lies in
lies in
![[formula]](E19b.gif) . Now Proposition 1.5 will apply as claimed
to show (b') implies (b). A similar change should be made in (c').
. Now Proposition 1.5 will apply as claimed
to show (b') implies (b). A similar change should be made in (c').
![[formula]](E05a.gif)
![[formula]](E19a.gif) of
a chain in
of
a chain in ![[formula]](E19b.gif) lies in
lies in
![[formula]](E19b.gif) . Now Proposition 1.5 will apply as claimed
to show (b') implies (b). A similar change should be made in (c').
. Now Proposition 1.5 will apply as claimed
to show (b') implies (b). A similar change should be made in (c').
![[formula]](E25a.gif) has the metric approximation property
has the metric approximation property
![[formula]](E26a.gif) should be
should be
![[formula]](E26b.gif)
![[formula]](E28a.gif)
![[formula]](E28b.gif)
![[formula]](E28c.gif)
![[formula]](E28d.gif)
![[formula]](E29a.gif)
![[formula]](E34a.gif)
![[formula]](E38a.gif)
![[formula]](E39a.gif)
![[formula]](E39b.gif)
![[formula]](E42a.gif)
![[formula]](E49a.gif) (the superscript is missing).
(the superscript is missing).
![[formula]](E50a.gif) (the set of rational numbers).
(the set of rational numbers).
![[formula]](E50b.gif) (the empty set).
(the empty set).
![[beta]](E52a.gif) should be
should be
![[alpha ']](E52b.gif)
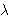 " should be "
" should be "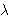
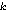 "
"