Inertially Expanding Clock

If B is controlled by the ticking of an inertially expanding clock, then these events are

$$(T,Z)=be^{n\Delta\tau} (1,0), ~~~~~~~n=n_1,n_2~.$$

These two events are marked by a square and a diamond in Figure 9. The constant $b$ is the proper time corresponding to $n=0$.

It follows from Eqs.(12) that the scattering event measured by B is

$${(t,z)=}$$

$$be^{\Delta\tau(n_1+n_2)/2}\left(\cosh \frac{n_2-n_1}{2}\Delta\tau ~,~ \sinh \frac{n_2-n_1}{2}\Delta\tau\right)$$

These three events, 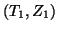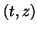$(T_1,Z_1)$, $(t,z)$ and $(T_2,Z_2)$, are marked in Figure 9 by a square, a circle, and a diamond.