Flow of Radiant T.E. Field Energy

Any loop antenna radiates only for a finite amount of time. Consequently, one can calculate the flow of total emitted energy, which is given by the spatial integral, Eq.(57). The fact that $\theta$ is a cyclic coordinate for axially symmetric sources and radiation implies that $\hat E_r=\hat B_\theta =0$ for the T.E. field. Consequently, the spatial integral, a conserved quantity independent of time $\xi$, reduces with the help of the table of derivatives in Section IV to

$$\int_{-\infty}^\infty \int_0^\infty \int _0^{2\pi} T^\xi_{~\tau}\,\xi d\tau \, rdr \,d\theta = \int_{-\infty}^\infty \int_0^\infty \int _0^{2\pi} \frac{1}{4\pi}\hat B_r \times\hat E_\theta \xi \,\xi d\tau \, rdr \,d\theta$$ (65)

$$= \frac{1}{4\pi}\int_0^\infty \int_0^\infty \int _0^{2\pi} \frac{1}... ...partial r} \frac{\partial \psi_F}{\partial \xi}\xi \,\xi d\tau \, rdr \,d\theta$$

$$= (\pm)\frac{(\pi a^2)^2}{\xi'^4_{I,II}}\int_{-\infty}^\infty \frac... ...u^3}\right) ^2 +\left( \frac{d^2q_{I,II}(\tau)}{d\tau^2}\right)^2 \right\}d\tau$$ (66)

The computation leading to the last line has been consigned to the Appendix. This computed quantity is the total energy flow (energy $\times$ velocity), or equivalently, the total momentum into the $\tau$-direction, radiated by a magnetic dipole accelerated uniformly in Rindler sector $I$ (upper sign) or in Rindler sector $II$ (lower sign).

The full scalar radiation field

$$\psi_F(\xi,\tau,r,\theta)=2\pi a^2 \frac{\pm 1}{\sqrt{(\xi^2-\xi^{'2}_I -r^2)^2+(2\xi \xi'_I)^2}} \frac{dq_{I,II}(\tau')}{d\tau}~,$$ (67)

with

$$\tau'=\tau \pm \sinh^{-1}\frac{\xi^2-\xi^{'2}_{I,II} -r^2}{2\xi \xi'_{I,II}}~,$$

is a linear functional correspondence. It maps the temporal history 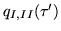$q_{I,II}(\tau')$ at $\xi'=\xi'_{I,II}$ in Rindler sector $I$ (resp. $II$) with 100% fidelity onto a readily measurable e.m. field along the $\tau$-axis (or a line parallel to it) on the spatial hypersurface $\xi=const.$ in Rindler sector $F$. 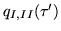This correspondence has 100% fidelity because, aside from a $\tau$-independent factor, the source history $q_{I,II}(\tau')$ and the scalar field $\psi_F(\tau)$ differ only by a constant $\tau$-independent shift on their respective domains $-\infty<\tau'<\infty$ and $-\infty<\tau<\infty$. This implies that

$$\frac{\partial}{\partial\tau'}=\frac{\partial}{\partial\tau}$$ (68)

when applied to the source function $q_{I,II}$. The expression for the radiated momentum becomes more transparent physically if one uses the proper time derivative

$$\frac{d}{dt'} \equiv \frac{1}{\xi'}\frac{\partial}{\partial\tau'} =\frac{1}{\xi'}\frac{\partial}{\partial\tau}$$

at the source. Introduce the (proper) magnetic moment of the current loop having radius $a$:

$$\textbf{m}=\pi a^2 \frac{1}{\xi'}\frac{\partial q}{\partial \tau'} ~.$$

One finds from Eq.(66) that the proper radiated longitudinal momentum (i.e. physical, a.k.a. orthonormal, component of energy flow pointing into the $\tau$-direction) measured per proper spatial $\tau$-interval $\xi\, d\tau$ in $F$ is

$${\mathcal I}_{T.E.}= (\pm)~\frac{\xi'^2}{\xi^2} \frac{2}{3}\... ...ac{1}{\xi'^2}\left(\frac{d \textbf{m}}{dt'}\right)^2 \right]~.$$ (69)

This is the formula for the proper radiant energy flow due to a magnetic dipole moment subject to uniform linear acceleration $1/\xi'$. There are two factors of $1/\xi$. The the first converts the coordinate $\tau$-momentum component into its physical component. The second is due to the fact that Eq.(69) expresses this quantity per proper distance into the $\tau$ direction.

When the acceleration $1/\xi'$ is small then the second term becomes small compared to the first. In fact, one recovers the familiar Larmor formula [#!Landau1962!#] relative to a static inertial frame by letting $\xi=\xi'$ and letting $1/\xi'\rightarrow 0$, which corresponds to inertial motion. By contrast, Eq.(69) is the correct formula for an accelerated dipole moment. However, observation of radiation from such a source entails that the measurements be made relative to an expanding inertial reference frame.