Application to Accelerated and Expanding Inertial Frames

A key virtue of splitting spacetime according to the 2+2 scheme is its flexibility. It accommodates the necessary Rindler coordinate geometries which are called for by the physical problem: accelerated frames for the accelerated sources, and expanding inertial frames for the inertial observers who measure the radiation emitted from these sources. These geometries are

$$ds^2 = -\xi^2 d\tau^2 +d\xi^2+dr^2 +r^2d\theta^2 \qquad \textrm{in}~I~\textrm{or~in}~II \quad \textrm{(\lq\lq accelerated ~frame'')}$$ (25)

and

$$ds^2 = -d\xi^2 +\xi^2 d\tau^2 +dr^2 +r^2d\theta^2 \qquad \textrm{in}~F~\textrm{or in}~P \quad\textrm{(\lq\lq expanding (or contracting) inertial frame'')}$$ (26)

In these two frames the Rindler/polar-coordinatized version of Eq.(5) is

$$\left[ \left( -\frac{1}{\xi^2} \frac{\partial ^2}{\partial ... ...u,\xi,r,\theta)~~~~~~~~~~~~ \textrm{in}~I~\textrm{or~in}~II~,$$ (27)

and

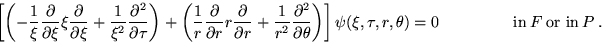$$\left[ \left( -\frac{1}{\xi} \frac{\partial}{\partial \xi}\... ...heta)=0~~~~~~~~~~~~~~~~~~~~~ \textrm{in}~F~\textrm{or~in}~P~.$$ (28)

Notational rule: The Rindler coordinates listed in the arguments of the scalar functions in Eqs.(27) and (28) are always listed with the timelike coordinate first, followed by the spatial coordinates. Thus $(\tau,\xi,r,\theta)$ implies that the function is defined on Rindler sectors $I$ or $II$, as in Eq.(27). On the other hand, $(\xi,\tau,r,\theta)$ implies that the domain of the function is $F$ or $P$, as in Eq.(28).

The feature common to the T.E. and the T.M. field is that both of them are based on the two-dimensional curl of a scalar, say $\psi$. The difference is that for the T.E. field this curl is in the Euclidean plane,

$$\nabla_a \times \psi \equiv \epsilon_{ab} ~^{(2)} g^{bc} \fr... ...\partial\theta}, -r \frac{\partial \psi}{\partial r} \right)~,$$

while for the T.M. field this curl is the Lorentz plane,

$$\nabla_A \times \psi \equiv \epsilon_{AB} ~^{(2)} g^{BC} \fr... ...{\partial\tau} \right)\quad \textrm{in }I \textrm{ or in }II~,$$

and

$$\nabla_A \times \psi \equiv \epsilon_{AB} ~^{(2)} g^{BC} \fr... ...}{\partial \xi} \right)\quad \textrm{in }F \textrm{ or in }P~,$$

The $\epsilon_{ab}$ and $\epsilon_{AB}$ are the components of the antisymmetric area tensors on the two respective planes.