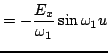Action-Angle Representation

In mechanics, periodicity, one of the most pervasive aspects of nature, leads to the introduction of action-angle variables as phase space coordinates for a dynamical system. One of the chief virtues of these variables is that they decompose the given dynamical system into its fundamental components, a set of noninteracting subsystems (``degrees of freedom'') each having its own frequency. This is why some mathematicians<sup>2</sup>, call these coordinates ``normal coordinates'', in analogy with the normal modes of a linear vibrating system.

Suppose the charged particle moves in the periodic electromagnetic field of a plane wave

$$A_x(u) = -\frac{E_x}{\omega_1} \sin\omega_1 u$$ (30)

$$A_y(u) = -\frac{E_y}{\omega_1} \sin(\omega_1 u+\delta)~,$$ (31)

which propagates along the positive $z$-direction with frequency be $\omega_1$. Then the vector potential, Eq.(13), satisfies

$$A_\mu(u+2\pi /\omega_1)=A_\mu (u)~,$$ (32)

and so does the superhamiltonian, Eq.(2).